Introduction
One of the most general definitions of creativity reads as follows: «Creativity is the process of solving a problem which gives rise to a new quality, and a new result» [Sosnin, 1997, p. 6; Beskova, 1993, p.162]. What does it mean to «creatively solve a problem», or to remove a contradiction? As we wrote earlier [Koblyakov, 2015, pp.261-279], the strategy of the computer in a problem-solving situation — in this case, the presence of mutually contradictory statements — comes down to a simple rejection of one of the alternatives, in other words, a reduction. In turn, the strategy of synthesis that combines alternatives is characteristic of a creative mind, which, at its core, differentiates it from a computer [Beskova, 1993, 165-169]. For example, Alfred Whitehead in his book «Process and Reality» (1929) writes that the purpose of art is «to move from disjunction to conjunction, and the formation of a new essence, different from the one given by disjunction» (Nalimov, 1989, p.162). Let us be reminded that in logic, the transition from disjunction to conjunction also means a transition from a disjoining statement to a conjoining one, from a juxtaposition or separation of qualities, processes, and phenomena, to a synthesis or a connection.
Conjunction Disjunction
Connection Statement Separation Statement
Figure 1.
In light of this idea, we can add to the previous definition of creativity, to clarify it using Whitehead’s logical component: Creation is a process of problem solving through the transition from disjunction to conjunction, with the birth of a new quality, and a new result (the synthesis of antitheses, forming a «new essence»). And in this transition from disjunction to conjunction, specifically, lies the very essence of creativity. So, how does this transition from disjunction to conjunction, from opposition to unity, occur? How do antagonists become partners, competitors become allies? Specifically, how does the synthesis of an antitheses occur? How does a new quality appear in the whole, which is absent in its parts? Referring back to the article [Koblyakov, 2015, 261-279].
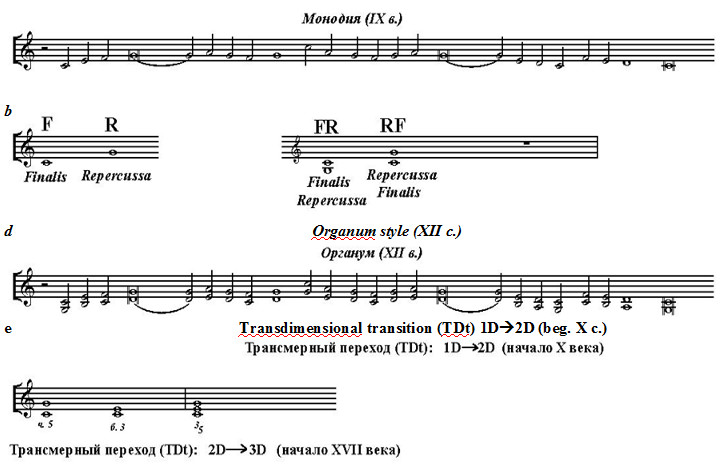
a Monody (IX c.)
Transdimensional transition (TDt) 2D3D (beg. XVII c.)
Figure 2 (a, b, c, d, e)
How is the synthesis of an antithesis in the simplest musical statement possible—a single voice melody? Lets look at pre-Baroque European monody (Fig. 2a). It is well known that this and similar chants have two types of foundations — the lower and upper stable tones («finalis» and «repercussa», see Fig. 2b). The finalis is the beginning and ending note of the chant, or the «harmonic tonic», while the repercussa is the «melodic tonic». In the initial case, the finalis and repercussa are juxtaposed as a disjunction. With the appearance of hypo modes, the functions of the finalis and repercussa are expanded in such a way that each of them takes on the quality of an antagonist: the finalis is presented by the middle tone (by the repercussa), while the repercussa is presented by the lower tone (by the finalis, see Fig. 2c). The next stage is a combination of the two: the same chant now sounds both from the finalis tone and repercussa tone SIMULTANIOUSLY. Which is more important- the finalis or the repercussa? Both have gained equal status, since they have coalesced into a simultaneously sounding harmonic fourth (fifth). The melody has been doubled: from each note of the fifth (fourth), now sounds the same melody (see Fig. 2d)! Disjunction has become conjunction, and the so-called “ Organum style” (X cen.) has come into being. What has occurred is a transition from single voice melodies to two voice melodies, from a single dimensional musical space (the building block of that which is the tone) to a dual dimensional space (the building block that is the interval). The new dimension provides a new parameter: consonance (the vertical coalescing of sounds); a new whole with a different quality has come into being, one which was absent in its initial parts. [The dimension of a space (system, element), refers to the degree of spatial freedom (system, element) which allows for both a geometric as well as a parametric (statistical) interpretation]. And so, the fundamental contradiction in a single voiced melodic line is resolved through the synthesis of antitheses in a two-voice melody. We then find that the entire history of music is a chain of such transitions. So, the next historical stage—the transition into a three-dimensional musical space that occurred in the 17th century—happened in the same manner. The fundamental problem of a two dimensional space was the contradiction between the interval of a fifth and the third (Fig. 2e, at the beginning of the 17th century, see more details [Koblyakov, 2015]). Now we understand the specific qualities of the transition from disjunction to conjunction: it is a special kind of transition — from a state of smaller dimensions to one of larger dimensions. Such a nontrivial quality of this transition demands that it be given its own term. We will call this transition a «transdimensional transition» (from the Latin root “trans”- meaning “through”, abbreviated TDt [Koblyakov, 2003, pp. 20-42].
From everything that has been said, it follows that precisely in this transition, from our point of view, lies the heart of the creative process as a transition from disjunction to conjunction, from opposition to unity, or, in general, a transition to greater degrees of freedom [Koblyakov, 2000a]. Let us point out once more that the resolution of the inherent contradiction through the synthesis of antitheses is possible only in a musical (semantic) meta-space relative to the initial one, i.e. only through a transdimensional transition into a multi-dimensional space (this assertion is logically proven in [Koblyakov, 2000b, pp.33-35]).
Now we can see the reason for our previous unsuccessful attempts at rationally describing the synthesis of antitheses: the disregard of different dimensional states of disjunction and conjunction. Conjunction is located at a higher dimension, than disjunction! Transition from disjunction to conjunction of – is special «transdimensional transition» (TDt). Transdimensional transition is a particular case of a new type of relationship that we have called «transdimensional relationship» or «transdimensionalism» (from the Latin root “trans” — meaning «through», abbreviated TD). Transdimensionalism refers to the entire set of relationships between spaces of differing dimensions within a multidimensional whole, i.e.: insertion, mutual reflection, folding-unfolding, connections between dimensions, transition from one dimension to another etc. (a few types of transdimensional relationships can be seen in Fig. 3).
Transdimensional transition (TDt) — a transition from one dimensional space to another dimensional space.
Polydimensionality (PD) — the simultaneous presence of an object in different dimensional spaces.
Isodimensionality (ID) — the presence of an object in the same dimensional space.
Transdimensional “cascade” (TDc) — the transition from one dimensional space to another, followed by a return to the initial dimension.
Variational dimension — a space with dimensional variation.
Transdimensional “cross” - the linking of a transdimensional horizontal line with a transdimensional vertical line.
Transdimensionalism- refers to the entire set of relationships between spaces of differing dimensions within a multidimensional whole, i.e.: insertion, mutual reflection, folding-unfolding, connections between dimensions, transition from one dimension to another etc.
Figure 3
To begin, the essence of creativity is in the transition from disjunction to conjunction, and in the synthesis of antitheses. A transition based on synthesis, giving birth to a new quality, is a special transdimentional transition from a semantic one — dimensional space to a space of different (higher) dimension. Precisely in a higher dimensional space (a meta space relative to the initial space) are the contradictions and paradoxes of the initial space eliminated, creating a new whole, which possesses new qualities. A transdimensional transition is an important special example out of an entire network of relations of a new type. We will call these relations «trandimensionalism» or «trandimentional relations». We will show their effectiveness in solving a whole row of traditionally difficult problems in the humanities and natural sciences.
Main part
How do language and speech relate to one another? Orlanguage units and speech units? To answer these questions we will need a different specific type of transdimensional relation. We are referring to «polydimensionality» (abbreviated PD) - which is the belonging of an object to spaces of different dimensions (see Fig.3). Let us begin once again with music. We will show that polydimensionality lies at the very heart of music — in the «simplest» modal unit (dyad) -»stable tone- unstable tone». Let us examine it more closely (or, using our terminology — let us perform its «transdimensional analysis»). To begin, the stable tone is independent from the unstable tone, it is defined unambiguously, and possesses a single degree of freedom. On the other hand, the unstable tone is dependent on the stable tone, and possesses two degrees of freedom: 1) it can be resolved into a stable tone, 2) or it can move away from it [Koblyakov, 2015]. In other words, from the point of view of musical grammar, an unstable tone is unambiguous, as is a stable tone. On the other hand, from the point of view of contextual meaning, a stable tone is bimodal, and is on the whole, two- dimensional. So, in the above pre-Baroque monody, the note C is a stable tone (finalis), it is the final note of a chant which has a single degree of freedom. Meanwhile, the unstable tone F (see Fig. 2a) has two degrees of freedom 1) it can be resolved into a stable tone (which is required by the rules of modal grammar, but happens only at the very end of the chant — in a downward movement), 2) or it can move away from this resolution (in this context, the tone F moves up to the tone G — see ex. 2). In this way, the dyad «stable tone-unstable tone» is polydimensional, one of its parts is one dimensional, while the other is polydimensional (PD = 1D: 2D). The polydimension 1D: 2D is the basic unit of transdimnsionality, allowingone to separate contextual meanings from acontextual meanings. From the above example it becomes clear that an unstable tone has an additional contextual meaning, as opposed to an acontextual stable tone. The space of a stable tone is an all encompassing space, which contains within it the space of a stable tone. Earlier, it was considered, that the dyad «stable tone- unstable tone» is the «simplest possible microstructure», a «melodic unit», and that its units have an equal status. Now it has become apparent that this is not the case: the «simplest» is in fact complex! In written form, a linear notation of this progression is inadequate: it obscures the polydimensionality (PD=1D:2D) of the right and left elements of the dyad «stable tone- unstable tone» [Koblyakov, 2015].
A trandimensional analysis of other binary structures in language produces the following results. Many binary pairs, similar to stable tone- unstable tone», are polydimensional, and have PD=1D:2D. From example: vibrator — resonator, action — reaction, action — reflection, horse — rider, carrier — carried, matter — information, etc. The second elements of these dyads are context-dependent, meaning, they are linked to the first ones, and moreover, can’t exist without them, as opposed to the first «autonomous», context-independent elements. So, a vibrator is self sufficient (context-independent), while a resonator requires the presence of a vibrator etc. [7]. It is precisely transdimensionality which allows us to differentiate simple binary pairs (oppositions) from complex ones: the deciding differentiating factor is the presence (or absence) of CONTEXT. All oppositions which contain noncontextual elements are simple (top- bottom, left- right etc.). Oppositions in which at least one element is context- dependent are complex (all the above opposition belong to this group). Context creates an «extra» (different) dimension, an additional (different) point of view, a new coordinate, degree of freedom etc. [Koblyakov, 2003].
Let us focus on this fact, in order to demonstrate the entire importance and necessity of transdimensional relations. As we know, binary oppositions are the basis of thought and language. They have been studied countless times by many scientists. However, no one has rationally described there main differentiating quality. Only now does the entire complexity of there fixation, the nonobvious nature of there differentiating qualities, the difficulty in separating simple oppositions from complex ones, become apparent. «Outside», in a horizontal projection, they are identical to one another, and are (following a geometric interpretation) «poles» (opposite points) of a single coordinate plane; on the other hand, «inside», in an invisible «depth», in a vertical projection, in complex oppositions (and only in complex ones), one of the points (one of the «poles») has a «font», a transition to a new dimension, which is absent from the other point- the «pole». In this way, the same object (a point, pole, or element of a system) is simultaneously located in two spaces of close dimension (one dimensional and two dimensional), which is the very essence of «polydimensionality». This «stratification», or «inner split» of an object is discovered by these new relations, allowing one to «see» through the depth of binary oppositions and their different qualities (polydimensionality), the depth and different qualities (polydimensionality) of language and thought itself.
Now we are ready to differentiate language from speech, language units from speech units. We will perform a transdimensional analysis of the pair «language-speech». After all that has been said above, the result is obvious. Language is autonomous, unambiguous, and context independent. Meanwhile, speech is «interpreted», «mediated» language, meaning that it is contextual, and does not exist without language. To put it simplified, language is unambiguous, its semantic space is one dimensional, while speed is ambiguous, its space is two dimensional. Obviously, the same relation of spatial dimensions (PD=1D:2D) exists between musical language and speech, and between any language and speech.
Let us compare units of language and speech. In musical language the progression «unstable tone-stable tone» will have a dimensionality of 1D — the same one that the overall semantic space has. The unstable tone will have a single, hard deterministic (and grammatically correct) trajectory of movement into the stable tone. In written form, this unambiguous progression is written in a linear form. On the other hand, in musical speech the unstable tone will have several options in its movement (and accordingly, also options of different stable tones). Instead of a single pair of «stable tone-unstable tone» we will have several pairs with different versions of resolution (or «zones»). So, in the monodic chant in figure 2a the note F, as was already pointed out, has several options in terms of its resolution. In written form, the options of resolution are, in this case, written in a non-linear form, in a two dimensional table with an x-axis and y-axis, which contains all the versions of the «answers» to the «questions» (resolutions of the unstable tone into different stable tones). In mathematics, such a table is called a «matrix».
And so, the transition from (musical) language to (musical) speech, which is so difficult to study, has occurred thanks to the transdimensional relations we have discovered, and specifically thanks to the example of polydimensionality. It is precisely polydimensionality which brought forth the key difference between language (a hard deterministic unambiguous system) and speech (an ambiguous version of language) as different dimensional states of a single whole. The units of language and speech relate to each other as element and matrix. At the same time, speech does not have its own structural units, instead reflecting units of one or another structural level of language each time, in a matrix form (in the form of versions-variants).
And how is creativity possible, and what are its preconditions? What plays the role of a «sky hook» in creative processes, in terms of our thinking? A transdimensional analysis allows us to answer this question, while bringing to light the incomplete nature of some ideas about our own thinking. Let us return, once again, to the musical dyad «stable tone-unstable tone», only this time we will examine it from the perspective of a linguistic space. Here it is also polydimensional. On the one hand, the elements of the dyad are equal and symmetrical, differentiated only by plus and minus signs (by «color»). On the other hand, the prefix «un» (in «unstable tone») creates a new quality (parameter): it not only negates the assertion («stable tone»), but has an unbreakable bond with it (it is not an overall negation, but a negation of this specific assertion!). As a result, the negation («unstable tone») is the complex element of the dyad, while the assertion («stable tone») is the simple element. From this perspective, the dyad «stable tone-unstable tone» is polydimensional (PD = 1D:2D) and asymmetrical. From this we can infer that all dyads which posses a negation are polydimensional and asymmetrical («truth — untruth»; «to be — not to be» etc.). The second (context- dependent) elements of these dyads create a pair, or a «link», with the first (acontextual, or free) element, which is pertinent to the study of thinking.
We have brought forth the incomplete nature of our ideas on the basis of thinking — binary oppositions, which, up to this point, were considered symmetrical. We have already shown above, that oppositions can be simple and complex (with a context- dependent element), and complex oppositions and asymmetrical! Let us be reminded, that this includes not only dyads with negation, but also (as has already been said), ones with a reflexive element (object — subject, action — observation), ones with secondary action (stimulus — reaction, vibrator — resonator), ones with synthesis in one of its elements (horse — rider) etc. All of these are examples of PD 1D:2D, or «transdimensional asymmetry», which has important logical ramifications. Firstly, acontextual terms are separated from contextual ones, this time, at the very first level: specifically, we find the, till now not obvious, polydimensionality of the logical dyads «yes-no», «true-false» etc. Secondly, when one takes into account transdimensional relations and polydimensionality, classical paradoxes cease to be paradoxes. For example, the «barber paradox» (Bertrand Russell): all the villagers are one dimensional, while the barber is polydimensional, PD = 1D: 2D. The king’s order does not take this into account, thus creating a paradox! (Same with the paradoxes «I am lying»; «I am asleep» etc). In other words, the paradoxes themselves are a sign of the inhomogeneity of linguistic spaces and thought spaces, and «suggest» a need for transdimensional relations and a new type of logic (a «logic of creation» [Koblyakov, 2000a, 2000b, 2003, 2015], uniting, through PD = 1D:2D, classical and nonclassical logic in one representation. Taking into account the transdimensionality of polydimensional logical spaces removes paradoxes entirely (rather then obscuring them, as in Russell’s hierarchy of types). But the most fundamental aspect — and the one, which contains the answer to the question above — is that polydimensionality (PD) brings to light the unique creative role of negation as an «unlocking» phenomenon, bringing the system «outside the barriers», into a higher dimension. It is negation, which creates the necessary preconditions of creativity, considering the fact that negation specifically lies at the basis of the contradiction which «launches» the creative processes, as we have shown. It through negation, that language norms and rules are called into question, leading to the problematisation of the initial statement. Moreover, the transdimensional transition from disjunction to conjunction becomes possible only with the presence of an «extra» degree of freedom, that is already present in the negation! The very possibility of negation is the precondition and the necessary condition of the creative process. It’s also worth pointing out, that this conclusion is in line with the findings of neurophysiology. It is considered, that the brain’s right hemisphere contains a person’s creative abilities, it is the location of the «creator», while the left contains the «doer». When placed under the conditions of paradoxical mind (when only the right hemisphere is functioning), a person indeed becomes «Mister NO»: he rejects (brings into doubt), any assertions, including even obvious ones! The «creative» hemisphere is based on negation. (And the opposite is also true: when only the left hemisphere is functioning, a person becomes «Mister YES»: he agrees with any assertion, no matter how absurd, and follows any rule and instruction).
Negation is tightly linked to falsehood. Falsehood and creativity often go together (especially when it comes to kids — such as children’s fantasy stories). There exists a well known thesis about child’s psychology: a child becomes an individual from the moment he learns to lie. This thesis can be proven. What is «individuality»? It is non other then the ability to create! If one is capable of lying, then one is also capable of fantasizing and creating! All the while, the polydimensionality of the dyad «truth-falsehood» is proven via experiment (through MRI scans of the brain). When a person is telling the truth, one area of the brain is activated, which deals with reality as it is; when he is lying, two areas are activated, dealing with both truth and falsehood! However, keeping two areas of the brain active simultaneously is not easy, and this is why very young children don’t lie («they are not yet individuals»).
The polydimensionality of the dyad «yes-no» expands our idea of thinking. But why are they still absent from the scientific thesaurus? One of the most important factors that lead them to be hidden from the eyes of scholars is the existence of the written word (text). The flatness of paper and the linear manner in which text is written does not allow one to discover the polydimensionality of structures! Whether it be dyads with negation, synthesis, secondary action, complex and reflexive opposition, etc — they are all written in a single linear form. We write the triad «thesis-antithesis-synthesis» as a one dimensional linear structure, even though synthesis is located in a different dimension plane relative to thesis! The same is true of the linear notation of the transition from disjunction to conjunction; it does not show the polydimensionality of the outer points of this transition, as we have already noted many time. Written language in its traditional form is not capable of showing transdimensional relations! It is based on text, which is, by definition, linear and moves in a single direction. Despite the constant attempts to free oneself from the «prison of linearity») (the works of Leroi-Gourhan, Derrida etc), text continues to obscure the polydimensionality of its elements through the practice of linear notation of elements in a chain of similar symbols. But the written language is, in reality, merely a reflection of out thinking, which means that we are talking about the incompleteness of our thinking as a whole! In transdimensionalism, there are resources which can eliminate this incompleteness! (It’s worth adding, that the development of computers and new hyper-textual technologies gives us hope for a more effective way of overcoming the «prison of linearity»).
Since it is precisely in artistic creation where we find relations, which are responsible for the meaning, goal, integrity, and value (of a work of art, see more [Koblyakov, 2015], this means that the creation of an artwork can become a new cognitive model in science, a new evolutionary standard model. It is with this model that all processes of creating the complex out of the simple, the whole from the part, will be correlated. In this way, the Subject will finally enter into the scientific worldview, in a strictly rational way. The consequences of this are «innumerable», and first and foremost in the area of scientific modeling. We are talking about a new type of modeling, that we believe will gain a new meaning in perspective — in addition to the existing mathematical modeling it will add «aesthetic modeling», as a new, higher level. So, how do they relate to one another?
We studies considered «harmony» to be a part of «algebra» earlier (mathematical modeling of the creative process). Now, the time has arrived for an inversion of the strategy of scientific study — from «harmony» to «algebra». Before this, works of art were not studied using the mathematics that was required for this, but only with that which was available! We were looking for it not «where it was lost», but «where there was more light» (mainly using statistical methods). In other words, ‘»harmony», came under the already existing «algebra». Now the situation has changed. A work of art is a new informative model, the value of which cannot be overstated. «Harmony» will become the customer, which orders a specific type of «algebra». The relations found in a work of art, which are responsible for the meaning, goal, integrity, and value of the artistic product are formulated in the universal language of logic and are then extrapolated unto other spheres of knowledge, first and foremost — unto mathematics. What kind of mathematics is necessary from the point of view of these new transdimensional relations? What type of «algebra» is more adequate for «harmony»? First, it must be process-based. Its objects are not set, but are created, including its space (the space of transdimensional transitions). We should remember that, say, the intervallic and chord spaces in musical evolution were not set, but were created! Had that not been the case, the categories of meaning, goal, and integrity would have remained outside the bounds of development… And how, for instance, can we represent the vector-dynamic shift from pitch to interval, and then to chords and polychords? Or how can we take the very same triad of «thesis-antithesis-synthesis», with its constantly changing dimensionality in the transitions from one element of the triad to another? What simplex corresponds to this dynamic structure? Because of this, all simplexes in this new mathematics must be dynamic, first of all, in terms of their dimensionality (a transdimensional space with transdimensional transitions; a transdimensional vector with shifting dimensionality; a transdimensional triangle with points of differing dimensionality etc). The category of «dimensionality» becomes a defining category in mathematics (which is understandable, given that any evolutionary processes are effective only with the presence of corresponding degrees of freedom), and from this we see an immediate need for it to be generalized in a Unified theory of Dimensionality. All of mathematics is thus divided into ID («iso-dimensional»)-mathematics and PD («poly-dimensional»)-mathematics (see Fig. 3). Modern science, which sees the world as language and text, naturally demands ID-mathematics; while the science of the future will see the world as speech and as a work of art, which will require PD-mathematics. It is worth pointing out, that the model being offered has nothing in common with creationism. It brings the Subject into our view of the world, which he (the Subject) creates from a strictly rationalistic perspective.
One of its most important innovations is methodological: it is an additional division of the Whole, this time not into levels (spaces of the same dimension), but into different dimensional spaces. The division of the whole according to «structural levels of matter» is now inadequate! Atoms, molecules, cells- these are all spaces of different dimensions (as are tones-intervals-chords inside a musical whole), they are not «levels» (of the same) three dimensional space. And so, in order to find the meaning, goal, and integrity of the phenomenon being studied, one must begin not with the whole space of higher dimension, but at the primal, basic one dimensional level — there one will find the roots of the problem, the key to all that follows!
How is all of this reflects on physics? Where, specifically, are transdimensional relations necessary in the future of physics? In our opinion, it is necessary, first of all, in the Grand Unified Field Theory. The theory has many different approaches that can be given priority. The new methodology allows us to select one, which is most promising. We can begin by pointing out, that a polydimensional whole consists of subspaces which are inside of it, and these spaces are not set, but are created as a response to a «challenge» — the problem at the basic one dimensional level, which moves from one space to another. In this way, each subsequent space of higher dimension «grows» out of the previous lower dimensional space (in music, intervallic space grows out of modal space, space chord grows out of intervallic space etc.). As a result, the spaces end up being inserted into one another through a limited principle, forming an indissoluble whole. Unlike a whole, in a conglomerate spaces are not created («grown»), but are set («taken in a ready state») a they are not linked by an organic insertion, and transdimensional relations are absent — this creates a loose mechanical whole. According to what principle are spaces in our universe linked, if it has such a «transdimensional vertical»? One of the Grand Unified Field Theories shows us in a fascinating way, that there is both transdimensionality and polydimensionality!